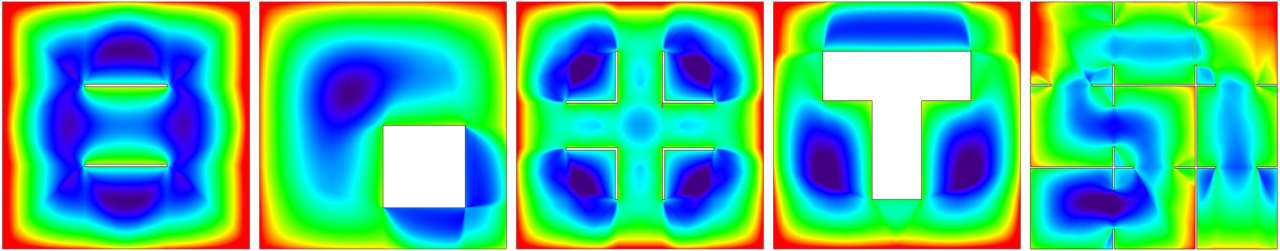
Variance (Tv) expresses the mean of the square of deviation between all radial lengths and average radial length of an isovist (Benedikt, 1979). In visibility graph terminology, it represents the point second moment for a location. Variance indicates both the complexity and eccentricity of an isovist. It takes other measures, studied simultaneously, to sort out which. For example, low Drift and high Variance will indicate complexity but not eccentricity (i.e. non centered-ness in one’s isovist).
To determine Variance at point ‘V’, the Isovist_App calculates the average distance between each edge of the polygon of the isovist, and the origin point of the isovist calculated at V. It then finds the square of the difference between the latter and Average Radial (Qv). It subsequently calculates the mean of all such values, as weighted by the arc angle associated to each edge. The final outcome is square rooted.
In notation form the calculation for Variance is expressed as:
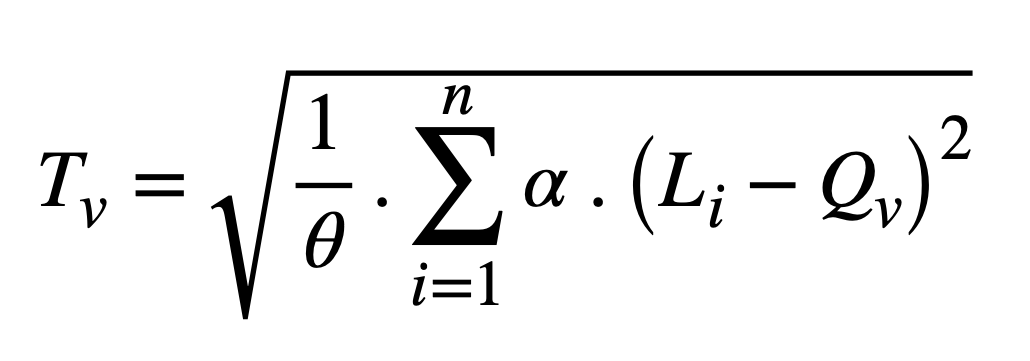
Where Li is is average length to each edge of the isovist polygon, Qv the average radial value, n the total number of edges of the isovist, alpha the angle subtended by each edge, and theta the total sweep of the isovist.